Diff for "CirrusZodiacal"

Differences between revisions 8 and 13 (spanning 5 versions)

Galactic Cirrus and Zodiacal Light

60K 50AU Dyson shell

\Large B(\lambda) = \LARGE { { 2 h c } \over { \lambda^3 \left( e^{ h c / \lambda k T } - 1 \right) } } = { { 4e19 ~ Jy / sr ~ {\mu}m^3 } \over { \lambda^3 \left( e^{ 240 {\mu} m / \lambda } - 1 \right) } }

This is for an infinite resolution imager - at large distances, the power is the image angular area divided by the pixel angular area. This scales the intensity way down for distant Dyson shells. If the ratio of shell intensity to background is F , the pixel size in arcseconds is px , the shell radius in AU is r , and the distance in parsecs is R , then the angular size A is:

A ~ = ~ \pi ( r / R )^2

F ~ = ~ px^2 / A = ( px ~ R / r ) ^2 / \pi

R ~ = ~ ( 50" / px ) \sqrt{ \pi F }

Detectability

px is 90" (90 arcseconds) for IRAS, 12" for WISE, and .11" for JWST. It is 2.3" for Spitzer MIPS (Multiband Imaging Photometer for Spitzer) 24 μm (128x128 pixels) and 9.4" for Spitzer 70 μm (16x32 pixels).

R ~=~ 0.96 \sqrt{ F } for IRAS, 7.2 \sqrt{ F } for WISE, and 790 \sqrt{ F } for JWST. Of those three, only IRAS has sensors for wavelengths longer than 25 μm. R ~=~ 38 \sqrt{ F } for 24 μm Spitzer and 9.2 \sqrt{ F } for 70 μm Spitzer.

Disk

Assume 3 pixels wide for identification of a disk. IRAS can "see" a 270" disk ( 100/270 = 0.37 pc away - nothing expected), WISE can see a disk (100/12 = 8.3 pc away ), etc:

	Pixel	Image
IRAS	90"	0.37
WISE	12"	2.8
Spitzer 24 μm	2.3"	14.5
JWST 25 μm	0.11"	300

from http://lambda.gsfc.nasa.gov/product/cobe/cobe_image_table.cfm

The graphs at the NASA site are not scaled, but they resemble logarithmic-scaled graphs in Kelsall, 1998, Figure 2. Units MJy/sr.

Color	Blue	Blue	Blue	Blue	Cyan	Cyan	Gren	Gren	Yelw	Yelw	Orng	Orng	Red	Red	Gray	Gray	Whit
4.9 μm	0.10	0.14	0.19	0.26	0.35	0.49	0.67	0.92	1.26	1.72	2.37	3.25	4.46	6.12	8.39	11.5	15.8
12 μm	1.58	2.02	2.58	3.29	4.21	5.37	6.86	8.77	11.2	14.3	18.3	23.3	29.8	38.1	48.7	62.2	79.4
25 μm	3.98	4.80	5.79	6.98	8.41	10.1	12.2	14.7	17.8	21.4	25.8	31.2	37.6	45.3	54.6	65.9	79.4
60 μm	1.00	1.31	1.73	2.27	2.99	3.92	5.16	6.78	8.91	11.7	15.4	20.2	26.6	35.0	46.0	60.4	79.4

The zodiac is highly inclined compared to the galaxy, but these plots show it intersecting near galactic center. This is fortunate if true - we are unlikly to distinguish a Dyson shell where the background is very dense (too confusing) or very thin (radially outwards, fewer candidates) - this puts two problem areas in the same place.

frequency = 299792 GHz-μm/λ
Ellis: Z zodiacal, C cirrus
B 60K 100 AU 1-sun Dyson, full pixel

Band(um)	12μm	25μm	60μm	70 μm	100μm
frequency	25 THz	12 THz	5 THz	4.3 THz	3 THz
Z nW/m²-sr	2800	1800	190	130	42
Z Jy-sr	1.12e7	1.5e7	3.8e6	3.0e6	1.4e6
C nW/m²-sr	4.8	2.3	3.2	4.5	13
C Jy-sr	1.9e4	1.9e4	6.4e4	1.1e5	4.3e5
B Jy-sr	4.8e7	1.7e11	3.5e12	3.9e12	4.0e12
B/(C+Z)	4.3	1.1e4	9.1e5	1.3e6	2.2e6	we may ignore zodiacal measurements
F=B/C	2.5e3	8.9e6	5.5e7	3.5e7	9.3e6	assume measurements in galactic plane
R IRAS pc	48	2900	7100	--	2900
R WISE pc	360	21000	--	--	--
R Spitzer pc	--	110000	--	54000	--
R JWST pc	40000	2400000	--	--	--

Question: In the very short wavelengths, does the presence of a shell block luminosity in a detectable way - a "round hole" in a uniform nebula?

-  ⇤ ← Revision 8 as of 2015-10-10 20:04:28 → 
  Size: 2697
  Editor: KeithLofstrom
  Comment:
+   ← Revision 13 as of 2015-10-15 07:54:21 → ⇥
  Size: 4595
  Editor: KeithLofstrom
  Comment:
-Deletions are marked like this.
+Additions are marked like this.
 Line 7:
-$ \Large B_{\nu}(\lambda) = \LARGE { { 2 h c } \over { \lambda^3 \left( e^{ h c / \lambda k T } - 1 \right) } } = { { 4e19 ~ Jy / sr ~ {\mu}m^3 } \over { \lambda^3 \left( e^{ 240 {\mu} m / \lambda } - 1 \right) } } $
+$ \Large B(\lambda) = \LARGE { { 2 h c } \over { \lambda^3 \left( e^{ h c / \lambda k T } - 1 \right) } } = { { 4e19 ~ Jy / sr ~ {\mu}m^3 } \over { \lambda^3 \left( e^{ 240 {\mu} m / \lambda } - 1 \right) } } $
 Line 15:
-$ R ~ = ~ ( 50 / px ) \sqrt{ \pi F } $
+$ R ~ = ~ ( 50" / px ) \sqrt{ \pi F } $
 Line 17:
-px is 90" (90 arcseconds) for IRAS, 12" for WISE, and .11" for JWST so
+-----
=== Detectability ===
-Line 19:
+Line 20:
-$ R ~ = ~ 0.98 \sqrt{  F } $ for IRAS, $ 2.7 \sqrt{ F } $ for WISE, and  $ 28 \sqrt{ F } $ for JWST.  However, only IRAS has sensors for wavelengths longer than 25 &mu;m.
+$px$ is 90" (90 arcseconds) for IRAS, 12" for WISE, and .11" for JWST.  It is 2.3" for Spitzer MIPS ('''M'''ultiband '''I'''maging '''P'''hotometer for '''S'''pitzer) 24 &mu;m (128x128 pixels) and 9.4" for Spitzer 70 &mu;m (16x32 pixels).
-Line 21:
+Line 22:
+$ R ~=~ 0.96 \sqrt{ F } $ for IRAS, $ 7.2 \sqrt{ F } $ for WISE, and  $ 790 \sqrt{ F } $ for JWST.  Of those three, only IRAS has sensors for wavelengths longer than 25 &mu;m.  $ R ~=~ 38 \sqrt{ F } $ for 24 &mu;m Spitzer and $ 9.2 \sqrt{ F } $ for 70 &mu;m Spitzer.

-----
=== Disk ===

Assume 3 pixels wide for identification of a disk.  IRAS can "see" a 270" disk ( 100/270 = 0.37 pc away - nothing expected),  WISE can see a disk (100/12 = 8.3 pc away ), etc:

||                  || Pixel || Image ||
|| IRAS             ||   90" ||  0.37 ||
|| WISE             ||   12" ||   2.8 ||
|| Spitzer 24 &mu;m ||  2.3" ||  14.5 ||
|| JWST 25 &mu;m    || 0.11" ||   300 ||
-Line 24:
+Line 37:
+from http://lambda.gsfc.nasa.gov/product/cobe/cobe_image_table.cfm
-Line 25:
+Line 39:
-WHAT IS THE SCALE??? from http://lambda.gsfc.nasa.gov/product/cobe/cobe_image_table.cfm
+The graphs at the NASA site are not scaled, but they resemble logarithmic-scaled graphs in Kelsall, 1998, Figure 2.  Units MJy/sr.
-Line 27:
+Line 41:
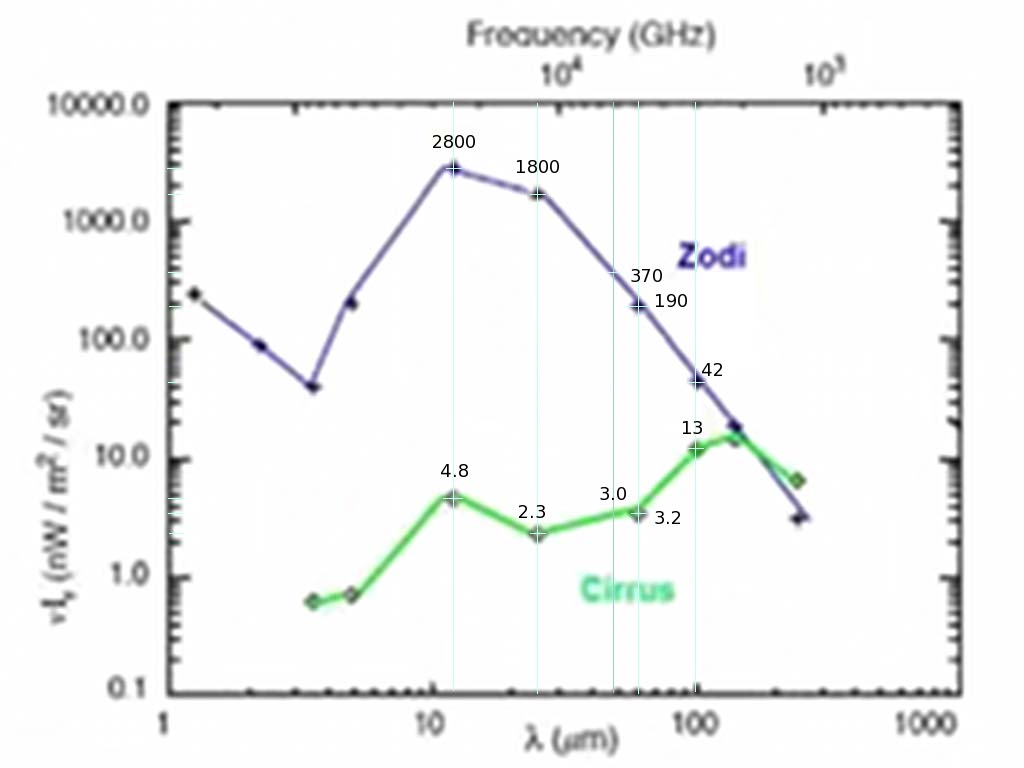
-The zodiac is highly inclined compared to the galaxy, but these plots show it intersecting near galactic center.  This is fortunate if true - we are unlikly to distinguish a Dyson shell where the background is very dense (too confusing) or very thin (radially outwards, fewer candidates).
+||Color || Blue|| Blue|| Blue|| Blue|| Cyan|| Cyan|| Gren|| Gren|| Yelw|| Yelw|| Orng|| Orng|| Red || Red || Gray|| Gray|| Whit||
||4.9 μm|| 0.10|| 0.14|| 0.19|| 0.26|| 0.35|| 0.49|| 0.67|| 0.92|| 1.26|| 1.72|| 2.37|| 3.25|| 4.46|| 6.12|| 8.39|| 11.5|| 15.8||
|| 12 μm|| 1.58|| 2.02|| 2.58|| 3.29|| 4.21|| 5.37|| 6.86|| 8.77|| 11.2|| 14.3|| 18.3|| 23.3|| 29.8|| 38.1|| 48.7|| 62.2|| 79.4||
|| 25 μm|| 3.98|| 4.80|| 5.79|| 6.98|| 8.41|| 10.1|| 12.2|| 14.7|| 17.8|| 21.4|| 25.8|| 31.2|| 37.6|| 45.3|| 54.6|| 65.9|| 79.4||
|| 60 μm|| 1.00|| 1.31|| 1.73|| 2.27|| 2.99|| 3.92|| 5.16|| 6.78|| 8.91|| 11.7|| 15.4|| 20.2|| 26.6|| 35.0|| 46.0|| 60.4|| 79.4||

The zodiac is highly inclined compared to the galaxy, but these plots show it intersecting near galactic center.  This is fortunate if true - we are unlikly to distinguish a Dyson shell where the background is very dense (too confusing) or very thin (radially outwards, fewer candidates) - this puts two problem areas in the same place.
-Line 33:
+Line 53:
- . D 60K 100 AU 1-sun Dyson, full pixel
+ . B 60K 100 AU 1-sun Dyson, full pixel
-Line 35:
+Line 55:
-|| Band(um)       ||  12&mu;m ||  25&mu;m ||  60&mu;m || 100&mu;m ||
|| frequency      ||   25 THz ||   12 THz ||    5 THz ||    3 THz ||
|| Z nW/m^2^-sr   ||     2800 ||     1800 ||      190 ||       42 ||
|| Z Jy-sr        ||   1.12e7 ||    1.5e7 ||    3.8e6 ||    1.4e6 ||
|| C nW/m^2^-sr   ||      4.8 ||      2.3 ||      3.2 ||       13 ||
|| C Jy-sr        ||    1.9e4 ||    1.9e4 ||    6.4e4 ||    4.3e5 ||
|| D Jy-sr        ||    4.8e7 ||   1.7e11 ||   3.5e12 ||   4.0e12 ||
|| D/(C+Z)        ||      4.3 ||    1.1e4 ||    9.1e5 ||    2.2e6 || we will probably ignore zodiacal measurements ||
|| F=D/C          ||    2.5e3 ||    8.9e6 ||    5.5e7 ||    9.3e6 || assume measurements in galactic plane ||
|| R IRAS     pc  ||       49 ||     2900 ||     7000 ||     3000 ||
|| R WISE     pc  ||      135 ||     8000 ||       -- ||       -- ||
|| R JWST     pc  ||     1400 ||    84000 ||       -- ||       -- ||
+|| Band(um)       ||  12&mu;m ||  25&mu;m ||  60&mu;m || 70 &mu;m || 100&mu;m ||
|| frequency      ||   25 THz ||   12 THz ||    5 THz ||  4.3 THz ||    3 THz ||
|| Z nW/m^2^-sr   ||     2800 ||     1800 ||      190 ||      130 ||       42 ||
|| Z Jy-sr        ||   1.12e7 ||    1.5e7 ||    3.8e6 ||    3.0e6 ||    1.4e6 ||
|| C nW/m^2^-sr   ||      4.8 ||      2.3 ||      3.2 ||      4.5 ||       13 ||
|| C Jy-sr        ||    1.9e4 ||    1.9e4 ||    6.4e4 ||    1.1e5 ||    4.3e5 ||
|| B Jy-sr        ||    4.8e7 ||   1.7e11 ||   3.5e12 ||   3.9e12 ||   4.0e12 ||
|| B/(C+Z)        ||      4.3 ||    1.1e4 ||    9.1e5 ||    1.3e6 ||    2.2e6 || we may ignore zodiacal measurements   ||
|| F=B/C          ||    2.5e3 ||    8.9e6 ||    5.5e7 ||    3.5e7 ||    9.3e6 || assume measurements in galactic plane ||
|| R IRAS      pc ||       48 ||     2900 ||     7100 ||       -- ||     2900 ||
|| R WISE      pc ||      360 ||    21000 ||       -- ||       -- ||       -- ||
|| R Spitzer   pc ||       -- ||   110000 ||       -- ||    54000 ||       -- ||
|| R JWST      pc ||    40000 ||  2400000 ||       -- ||       -- ||       -- ||


Question:  In the very short wavelengths, does the presence of a shell '''''block''''' luminosity in a detectable way - a "round hole" in a uniform nebula?