CirrusZodiacal

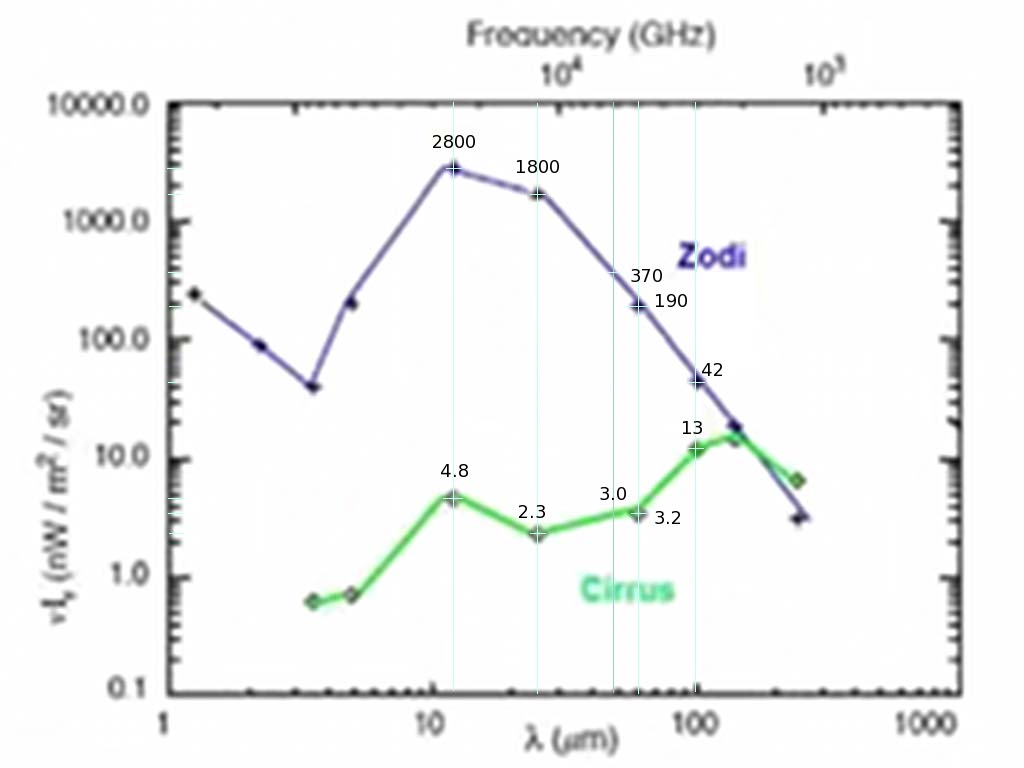
Galactic Cirrus and Zodiacal Light

60K 50AU Dyson shell

\Large B_{\nu}(\lambda) = \LARGE { { 2 h c } \over { \lambda^3 \left( e^{ h c / \lambda k T } - 1 \right) } } = { { 4e19 ~ Jy / sr ~ {\mu}m^3 } \over { \lambda^3 \left( e^{ 240 {\mu} m / \lambda } - 1 \right) } }

This is for an infinite resolution imager - at large distances, the power is the image angular area divided by the pixel angular area. This scales the intensity way down for distant Dyson shells. If the ratio of shell intensity to background is F , the pixel size in arcseconds is px , the shell radius in AU is r , and the distance in parsecs is R , then the angular size A is:

A ~ = ~ \pi ( r / R )^2

F ~ = ~ px^2 / A = ( px ~ R / r ) ^2 / \pi

R ~ = ~ ( 50 / px ) \sqrt{ \pi F }

px is 90" (90 arcseconds) for IRAS, 12" for WISE, and .11" for JWST so

R ~ = ~ 0.98 \sqrt{ F } for IRAS, 2.7 \sqrt{ F } for WISE, and 28 \sqrt{ F } for JWST. However, only IRAS has sensors for wavelengths longer than 25 μm.

WHAT IS THE SCALE??? from http://lambda.gsfc.nasa.gov/product/cobe/cobe_image_table.cfm

The zodiac is highly inclined compared to the galaxy, but these plots show it intersecting near galactic center. This is fortunate if true - we are unlikly to distinguish a Dyson shell where the background is very dense (too confusing) or very thin (radially outwards, fewer candidates).

• frequency = 299792 GHz-μm/λ

• Ellis: Z zodiacal, C cirrus
• D 60K 100 AU 1-sun Dyson, full pixel