Path Attenuation due to Orbital and Latitude Elevation
|
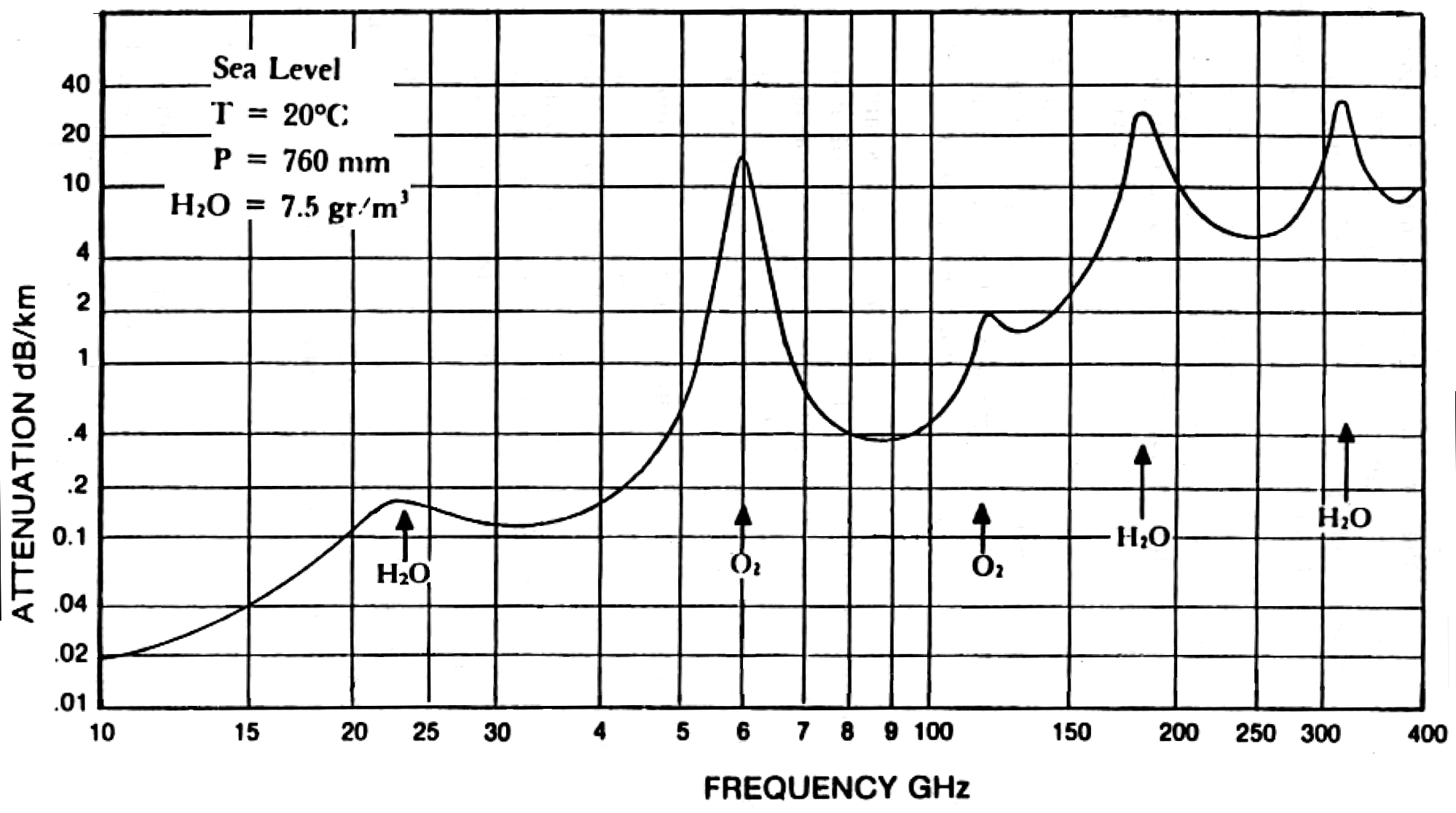
The atmosphere attenuates microwaves. This graph is from FCC bulletin 70, Millimeter Wave Propagation: Spectrum Management Implications , July 1997. |
The effective depth of the atmosphere ( Sea level pressure divided by sea level density and gravity ) is 8.5km, and microwaves arriving from space at an elevation angle of E travel through 8.5 / cos(E) kilometers of atmosphere. The attenuation trends upwards with frequency, with absorption peaks at frequencies where oxygen or water molecules resonate. The attenuation at 70GHz is 0.79dB/km, so the attenuation is 6.32 dB / cos(E), a factor of 4.29 at zenith (E = 90°) and 17.8 at 30° elevation.
Mountainous regions will have blocked visibility near the horizon, so fewer arrays will be in view. Higher altitudes mean lower attenuation; Kathmandu 28° N and 1400 meter altitude has 85% of sea level air density, so the attenuation is less than the above example, 5.37 dB / cos(E). For zero azimuth, E is 32 degrees, so the attenuation is 6.33 dB, the same as zero altitude on the equator.
Path attenuation versus latitude, zero azimuth
|
|
relative |
path length |
latitude |
elevation |
attenuation |
(km) |
0 |
90.00 |
1.00 |
6418 |
5 |
80.07 |
1.04 |
6466 |
10 |
70.36 |
1.16 |
6608 |
15 |
61.04 |
1.40 |
6837 |
20 |
52.24 |
1.82 |
7143 |
25 |
44.00 |
2.60 |
7514 |
30 |
36.34 |
4.16 |
7939 |
35 |
29.23 |
7.88 |
8406 |
40 |
22.62 |
19.75 |
8906 |
45 |
16.46 |
85.59 |
9430 |
50 |
10.69 |
1436.42 |
9970 |
Find thinsat density versus elevation
Note - At 170 GHz, that looks like 26dB/km. Through 7 equivalent kilometers of troposphere, that is about 180 dB attenuation at zenith. The stratosphere is dry and thin, so receivers at that height will absorb essentially all energy broadcast from space.
RECOMMENDATION ITU-R P.618-6 PROPAGATION DATA AND PREDICTION METHODS REQUIRED FOR THE DESIGN OF EARTH-SPACE TELECOMMUNICATION SYSTEMS
Satellite Communication Fundamentals Kadish and East Artech 2000 chapter 11 (have)