|
Size: 12889
Comment:
|
← Revision 31 as of 2021-06-18 19:23:36 ⇥
Size: 12791
Comment:
|
| Deletions are marked like this. | Additions are marked like this. |
| Line 4: | Line 4: |
| We can minimize night light pollution, and advance perigee against light pressure orbit distortion, by turning the thinsat as we approach eclipse. The overall goal is to perform 1 complete rotation of the thinsat per orbit, with it perpendicular to the sun on the day-side of the earth, but turning it by varying amounts on the night side. | We can minimize '''night light pollution (NLP)''' by turning the thinsat as we approach eclipse. The goal will be to perform one complete rotation of the thinsat per orbit, with it perpendicular to the sun on the day-side of the earth, but turning it by varying amounts on the night side. |
| Line 8: | Line 8: |
| == Maneuvering thrust and satellite power == | == Maneuvering Thrust and Satellite Power == |
| Line 20: | Line 20: |
| If things go very wrong, thinsats will be out of control and tumbling. In the long term, the uncontrolled thinsats will probably orient flat to the orbital plane, and reflect very little light into the night sky, but in the short term (less than decades), they will be oriented in all directions. This is equivalent to mapping the reflective area of front and back (2 π R^2^ ) onto a sphere ( 4 π R^2^ ). Light with intensity I shining onto a sphere of radius R is evenly reflected in all directions uniformly. So if the sphere intercepts π R^2^ I units of light, it scatters e I R^2^/4 units of light (e is albedo) per steradian in all directions. While we will try to design our thinsats with low albedo ( high light absorption on the front, high emissivity on the back), we can assume they will get sanded down and more reflective because of space debris, and they will get broken into fragments of glass with shiny edges, adding to the albedo. Assume the average albedo is 0.5, and assume the light scattering is spherical for tumbling. | If things go very wrong, thinsats will be out of control and tumbling. In the long term, the uncontrolled thinsats will probably orient flat to the orbital plane, and reflect very little light into the night sky, but in the short term (less than decades), they will be oriented in all directions. This is equivalent to mapping the reflective area of front and back (2 π R^2^ ) onto a sphere ( 4 π R^2^ ). Light with intensity I shining onto a sphere of radius R is evenly reflected in all directions uniformly. So if the sphere intercepts π R^2^ I units of light, it scatters e I R^2^/4 units of light (e is albedo) per steradian in all directions. While we will try to design our thinsats with low albedo ( high light absorption on the front, high emissivity on the back), we can assume they will get sanded down and more reflective because of space debris, and they will get broken into fragments of aluminum with shiny edges, adding to the albedo. Assume the average albedo is 0.5, and assume the light scattering is spherical for tumbling. |
| Line 22: | Line 22: |
| <<EmbedObject(g400.swf,play=true,loop=true,width=768,height=225)>> | {{ attachment:g400.png | | height=225 }} |
| Line 30: | Line 30: |
| === Max Night Light Pollution (NLP): Full power night sky coverage, maximum night light pollution === | === Max NLP: Full power night sky coverage, maximum night light pollution === |
Night Side Maneuvers
We can minimize night light pollution (NLP) by turning the thinsat as we approach eclipse. The goal will be to perform one complete rotation of the thinsat per orbit, with it perpendicular to the sun on the day-side of the earth, but turning it by varying amounts on the night side.
Another advantage of the turn is that if thinsat maneuverability is destroyed by radiation or a collision on the night side, it will come out of night side with a slow tumble that won't be corrected. The passive radar signature of the tumble will help identify the destroyed thinsat to other thinsats in the array, allowing another sacrificial thinsat to perform a "rendezvous and de-orbit". If the destroyed thinsat is in shards, the shards will tumble. The tumbling shards ( or a continuously tumbling thinsat ) will eventually fall out of the normal orbit, no longer get J_2 correction, and the thinsat orbit will "eccentrify", decay, and reenter. This is the fail-safe way the arrays will reenter, if all active control ceases.
Maneuvering Thrust and Satellite Power
Neglecting tides, the synodic angular velocity of the m288 orbit is \Large\omega = 4.3633e-4 rad/sec = 0.025°/s. The angular acceleration of a thinsat is 13.056e-6 rad/sec2 = 7.481e-4°/s2 with a sun angle of 0°, and 3.740e-4°/s2 at a sun angle of 60°. Because of tidal forces, a thinsat entering eclipse will start to turn towards sideways alignment with the center of the earth; it will come out of eclipse at a different velocity and angle than it went in with.
If the thinsat is rotating at \omega and either tangential or perpendicular to the gravity vector, it will not turn while it passes into eclipse. Otherwise, the tidal acceleration is \ddot\theta = (3/2) \omega^2 \sin 2 \delta where \delta is the angle to the tangent of the orbit. If we enter eclipse with the thinsat not turning, and oriented directly to the sun, then \delta = 30° .
Three Strategies and a Worst Case Failure Mode
There are many ways to orient thinsats in the night sky, with tradeoffs between light power harvest, light pollution, and orbit eccentricity. If we reduce power harvest, we will need to launch more thinsats to compensate, which makes more problems if the system fails. I will present three strategies for light harvest and nightlight pollution. The actual strategies chosen will be blend of those.
Tumbling
If things go very wrong, thinsats will be out of control and tumbling. In the long term, the uncontrolled thinsats will probably orient flat to the orbital plane, and reflect very little light into the night sky, but in the short term (less than decades), they will be oriented in all directions. This is equivalent to mapping the reflective area of front and back (2 π R2 ) onto a sphere ( 4 π R2 ). Light with intensity I shining onto a sphere of radius R is evenly reflected in all directions uniformly. So if the sphere intercepts π R2 I units of light, it scatters e I R2/4 units of light (e is albedo) per steradian in all directions. While we will try to design our thinsats with low albedo ( high light absorption on the front, high emissivity on the back), we can assume they will get sanded down and more reflective because of space debris, and they will get broken into fragments of aluminum with shiny edges, adding to the albedo. Assume the average albedo is 0.5, and assume the light scattering is spherical for tumbling.
Source for the above animation: g400.c
Three design orientations
All three orientations shown are oriented perpendicularly in the daytime sky. Max remains perpendicular in the night sky, Min is oriented vertically in the night sky, and Zero is edge on to the terminator in the night sky. All lose orientation control and are tilted by tidal forces in eclipse - the compensatory thrusts are not shown. Min and Zero are accelerated into a slow turn before eclipse, so they come out of the eclipse in the correct orientation. In all cases, there will probably be some disorientation and sun-seeking coming out of eclipse, until each thinsat calibrates to the optimum inertial turn rate during eclipse. So, there may be a small bit of sky glow at the 210° position, directly overhead at 2am and visible in the sky between 10pm and 6am.
Max NLP: Full power night sky coverage, maximum night light pollution
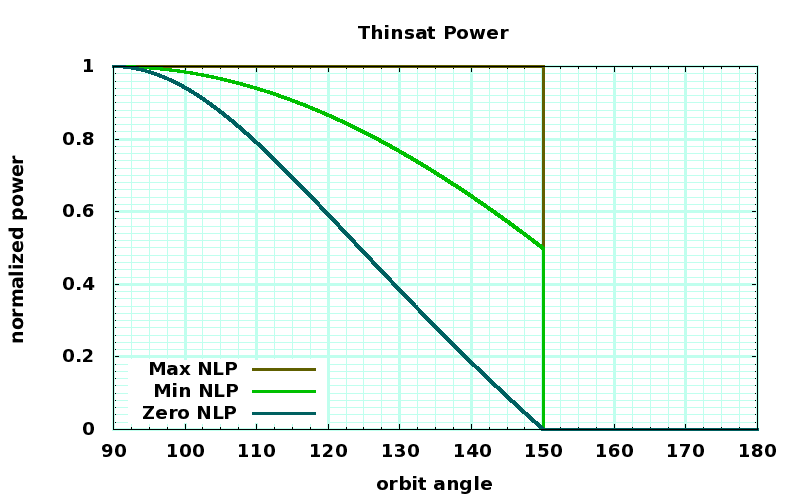
The most power is harvested if the thinsats are always oriented perpendicular to the sun. During the half of their orbit into the night sky, there will be some diffuse reflection to the side, and some of that will land in the earth's night sky. The illumination is maximum along the equator. For the M288 orbit, about 1/6th of the orbit is eclipsed, and 1/2 of the orbit is in daylight with the diffuse (Lambertian) reflection scattering towards the sun and onto the day side of the earth. Only the two "horns" of the orbit, the first between 90° and 150° (6pm to 10pm) and the second between 210° and 270° (2am to 6am) will reflect night into the light sky. The light harvest averages to 83% around the orbit.
This is the worst case for night sky illumination. Though it is tempting to run thinsats in this regime, extracting the maximum power per thinsat, it is also the worst case for eccentricity caused by light pressure, and the thinsats must be heavier to reduce that eccentricity.
Min NLP: Partial night sky coverage, some night light pollution
This maneuver will put some scattered light into the night sky, but not much compared to perpendicular solar illumination all the way into shadow. In the worst case, assume that the surface has an albedo of 0.5 (typical solar cells with an antireflective coating are less than 0.3) and that the reflected light is entirely Lambertian (isotropic) without specular reflections (which will all be away from the earth). At a 60° angle, just before shadow, the light emitted by the front surface will be 1366W/m2 × 0.5 (albedo) × 0.5 ( cos 60° ), and it will be scattered over 2π steradians, so the illumination per steradian will be 54W/m2-steradian just before entering eclipse.
Estimate that the light pollution varies from 0W to 54W between 90° and 150° and that the average light pollution is half of 54W, for 1/3 of the orbit. Assuming an even distribution of thinsat arrays in the constellation, that is works out to an average of 9W/m2-steradian for all thinsats in M288 orbit.
The full moon illuminates the night side of the equatorial earth with 27mW/m2 near the equator. A square meter of thinsat at 6400km distance produces 9W/64000002 or 0.22 picowatts per m4 times the area of all thinsats. If thinsat light pollution is restricted to 5% of full moon brightness (1.3mW/m2), then we can have 6000 km2 of thinsats up there, at an average of 130 W/m2, or about 780GW of thinsats at m288. That is about a million tons of thinsats.
The orientation of the thinsat over a 240 minute synodic m288 orbit at the equinox is as follows, relative to the sun:
time min |
orbit degrees |
rotation rate |
sun angle |
Illumination |
Night Light |
0 to 60 |
0° to 90° |
0 ~ \Large\omega |
0° |
100% |
0W |
60 to 100 |
90° to 150° |
1 ~ \Large\omega |
0° to 60° |
100% to 50% |
0W to 54W |
100 to 140 |
150° to 210° |
4 ~ \Large\omega |
60° to 300° |
Eclipse |
0W |
140 to 180 |
210° to 270° |
1 ~ \Large\omega |
300° to 0° |
50% to 100% |
54W to 0W |
180 to 240 |
270° to 0° |
0 ~ \Large\omega |
0° |
100% |
0W |
The angular velocity change at 0° takes 250/7.481 = 33.4 seconds, and during that time the thinsat turns 0.42° with negligible effect on thrust or power. The angular velocity change at 60° takes 750/3.74 = 200.5 seconds, and during that time the thinsat turns 12.5°, perhaps from 53.7° to 66.3°, reducing power and thrust from 59% to 40%, a significant change. The actual thrust change versus time will be more complicated (especially with tidal forces), but however it is done, the acceleration must be accomplished before the thinsat enters eclipse.
The light harvest averages 78% around the orbit.
Zero NLP: Partial night sky coverage, no night light pollution
In this case, in the night half of the sky the edge of the thinsat is always turned towards the terminator. As long as the thinsats stay in control, they will never produce any nighttime light pollution, because the illuminated side of the thinsat is always pointed away from the night side of the earth. The average illumination fraction is around 68%.
The orientation of the thinsat over a 240 minute synodic m288 orbit at the equinox is as follows, relative to the sun:
time min |
orbit degrees |
average rotation rate |
sun angle |
Illumination |
Night Light |
0 to 60 |
0° to 90° |
0 \Large\omega |
0° |
100% |
0W |
60 to 100 |
90° to 150° |
1.5 \Large\omega |
0° to 90° |
100% to 0% |
0W |
100 to 140 |
150° to 210° |
3 \Large\omega Start at 3.333\Large\omega |
90° to 270° |
Eclipse |
0W |
140 to 180 |
210° to 270° |
1.5 \Large\omega |
270° to 0° |
0% to 100% |
0W |
180 to 240 |
270° to 0° |
0 \Large\omega |
0° |
100% |
0W |
Pedants and thinsat programmers take note: The actual synodic orbit period is 240 minutes and 6.57 seconds long; that results in 2190.44 rather than 2191.44 sidereal orbits per year, accounting for the annual apparent motion of the sun around the sky.
The light harvest averages 67% around the orbit.
Why would a profit maximizing operator settle for 67% when 83% was possible? Infrared-filtering thinsats reduce launch weight and can use the Zero NLP flip to increase the minimum temperature of a thinsat during eclipses. An IR filtering thinsat in maximum night light pollution mode will have the emissive backside pointed at 2.7K space when it enters eclipse; the thinsat temperature will drop towards 20K if it cannot absorb the 64W/m2 of 260K black body radiation reaching it from the earth through the 3.5μm front side infrared filter. The thinsat will become very brittle at those temperatures, and the thermal shock could destroy it. If the high thermal emissivity back side is pointed towards the 260K earth, the temperature will drop to 180K - still challenging, but the much higher thermal mobility may heal atomic-scale damage.
Details of the Zero NLP maneuver
In the night sky, assuming balanced thrusters, only tidal forces act on the thinsat, \ddot\theta = -(3/2) \omega^2 \sin( 2 \theta . Integrating numerically from the proper choice of initial rotation rate (10/9ths the average, due to tidal decceleration) we go from 30 degrees "earth relative tilt" at the beginning of eclipse, to 150 degrees tilt at the end of eclipse, with the night sky sending 260K infrared at the back side throughout the manuever. The entire disk of the earth is always in view, and always emits the same amount of infrared to a given radius, but the Lambertian angle of absorption changes.
Power versus angle
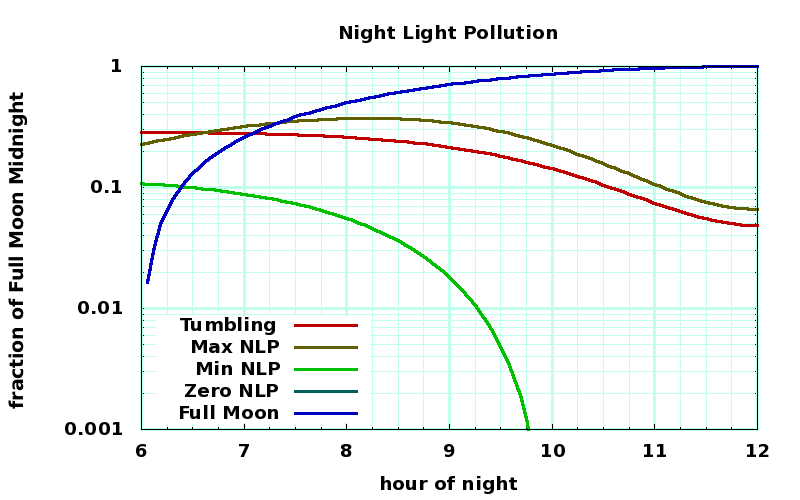
Night light pollution versus hour
The night light pollution for 1 Terawatt of thinsats at M288. Mirror the graph for midnight to 6am. Some light is also put into the daytime sky (early morning and late afternoon), but it will be difficult to see in the glare of sunlight. The Zero NLP option puts no light in the night sky, so that curve is far below the bottom of this graph.
Source for the above two graphs: nl02.c
Note to Astronomers
Yes, we will slightly occult some of your measurements, though we won't flash your images like Iridium. We will also broadcast our array ephemerides, and deliver occultation schedules accurate to the microsecond, so you can include that in your luminosity calculations. Someday, server sky is where you will perform those calculations, rather than in your own coal powered (and haze producing) computer.
